Rule of 70
What is the Rule of 70?
The Rule of 70 is a simple mathematical formula used to calculate the approximate time required for a quantity, growing at a constant rate, to double in size. It is also referred to as the ‘doubling time formula’ as it provides a useful ballpark estimate of the time it takes for a variable growing at a constant rate to double.
The Rule of 70 Formula
The formula for doubling time, as encapsulated by the Rule of 70, is expressed as:
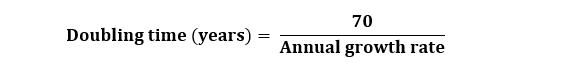
Hence, the doubling time is simply 70 divided by the constant annual growth rate. For instance, consider a quantity that grows consistently at 5% annually. According to the Rule of 70, it will take 14 years (70/5) for the quantity to double.
The Rule of 70 extends to contexts involving negative growth rates. In such cases, the formula helps estimate the time required for a quantity to halve, as opposed to doubling.
For instance, consider a quantity decreasing by a constant annual rate of 2%. Utilizing the Rule of 70:
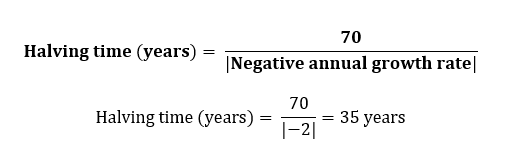
This implies that, under a sustained -2% growth rate, the quantity would shrink to half its current size in approximately 35 years (70/2).
The rule of 70 is derived from the properties of exponential growth. As such, the doubling time formula is used for compound interest rate as opposed to simple interest rate computations.
When growth is consistent, the rule provides a quick and easy way to estimate doubling time without the need for complex calculations. It is a close approximation to the more detailed logarithmic calculations used for this purpose.
Doubling Times: Actual vs. Rule of 70 Estimates
The table compares the actual and estimated doubling times using the Rule of 70 and the percentage variation between the two for a range of annual growth rates from 0.25% to 20%.
The percentage variation is calculated as the difference between the actual and estimated doubling times, as a percentage of the actual doubling time.
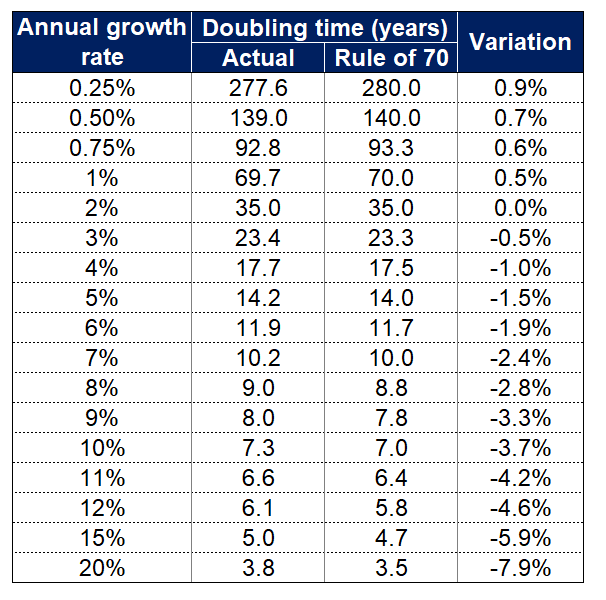
At lower growth rates (up to 4%), the estimated doubling times using the Rule of 70 are very close to the actual doubling times, with the variation being less than 1%. At a 2% growth rate, both the actual and estimated doubling times are 35 years, resulting in a 0% variation.
However, as the growth rate increases beyond 4%, the estimated doubling times from the Rule of 70 start to deviate more significantly from the actual doubling times, and the variation becomes increasingly negative.
This indicates that the Rule of 70 increasingly underestimates the doubling time as the growth rate increases. For example, at a 20% growth rate, the estimated doubling time is 3.5 years, whereas the actual doubling time is 3.8 years, resulting in a -7.9% (3.5/3.8 – 1) variation.
Rule of 70 vs. Rule of 72
The Rule of 70 and the Rule of 72 are essential tools in finance for estimating an investment’s doubling time. Both involve dividing a fixed number (70 or 72) by the compounded annual growth rate (CAGR) to approximate the number of periods, typically years, required for an investment to double.
The Rule of 70, while generally more accurate, is less convenient for mental calculations due to the indivisibility of 70 by common numbers such as 3, 4, 6, 8, 9, or 12. Conversely, the Rule of 72, being divisible by those numbers, is often preferred for its ease of use despite being slightly less accurate.
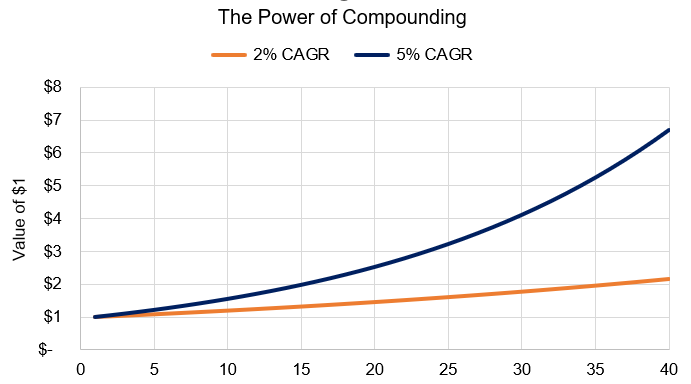
Graphical Illustration
The chart provides a graphical representation of the Rule of 70. On the x-axis, we have the annual growth rate, while the y-axis shows the doubling time in years.

A closer examination of the chart reveals an inverse relationship between the growth rate and the doubling time. As the annual growth rate (on the x-axis) increases, the time it takes for an investment to double (on the y-axis) decreases. To put this into perspective: a 10% growth rate sees a quantity double in approximately 7 years (70÷10), whereas at a 5% growth rate, this period stretches to around 14 years (70÷5).
A noteworthy observation is the pronounced decline in doubling time when the growth rate ranges between 1% and 10%. Beyond the 10% mark, the curve starts to flatten out, indicating diminishing returns in terms of reduction in doubling time with further increases in the growth rate.
Real-World Examples
The Rule of 70 can be applied in various fields, from finance and economics to demographics. In this section, we delve into diverse applications of the Rule of 70, highlighting its broad relevance through compelling examples.
Finance
In finance, the Rule of 70 can be applied to estimate the number of years it takes for investments to double, given a fixed annual growth rate. Consider this scenario: An individual places a sum in a bank offering a fixed annual interest rate of 2%. Using the Rule of 70, one can quickly deduce that it will be approximately 35 years (70 ÷ 2) before this deposit doubles in value.
In a more aggressive investment avenue, suppose an individual chooses to invest in an S&P 500 exchange-traded fund (ETF), which yields a consistent net total annual return of 7%. Using the Rule of 70, the investment would take approximately 10 years (70 ÷ 7) to double in value.
Economic Growth
In economics, the Rule of 70 provides a convenient rule of thumb to estimate the time it would take for a country’s real Gross Domestic Product (GDP) to double, given a constant real GDP growth rate. For instance, if Japan’s economy grows at a steady 0.5% each year, the rule suggests it will take 140 years (70/0.5) for the size of the economy to double.
In contrast, if Germany’s economy grows at 1.2% annually, it would only take about 58 years (70/1.2) for the size of its economy to double. The key takeaway here is that small differences in annual growth rates can result in significant differences in the size of economies over time due to the power of compounding.
Inflation
Inflation refers to the rate at which the general level of prices for goods and services is increasing. It erodes the purchasing power of money, as the same amount of money can buy fewer goods and services over time. The rule of 70 helps estimate how long it will take for a currency’s purchasing power to halve, assuming a constant annual inflation rate.
For instance, with a steady 3.5% annual inflation rate in the United States, the rule suggests that the US Dollar’s value will halve in about 20 years (70/3.5). Hence, if a basket of goods or services costs you US $100 today, in two decades, due to inflation, the price would rise to around US $200 for that same basket.
Population
In demographics, the Rule of 70 is useful for estimating the doubling time of a country’s population under the assumption of a constant rate of growth. For instance, if India’s forecasted growth rate is set at a steady 1.4%, the population is expected to double in approximately 50 years (70/1.4).
In Japan, if the annual population growth is set to shrink by 0.9% annually, the Rule of 70 formula estimates that the population will halve in 78 years (70/0.9).
Limitations of the Rule of 70
The Rule of 70 is predicated on a constant growth rate assumption. In reality, however, financial and economic variables such as interest rates, investment returns, inflation rates, and economic growth rates fluctuate. As such, variability in the growth rates can compromise the accuracy of the Rule of 70’s estimates.
The Rule of 70 is a linear approximation of an exponential growth function. Therefore, its result should be viewed as a rough estimate rather than a precise calculation. The Rule of 70 is more precise for annual rates that hover between 0.5% and 10% and tends to be increasingly less accurate for rates outside this range. Notably, for growth rates above 10%, the Rule of 70 underestimates the doubling time.
The Rule of 70 does not factor in the impact of different compounding periods, such as monthly or quarterly compounding. The rule is fundamentally based on the assumption of annual compounding when calculating doubling times.
However, in reality, the compounding frequency can vary, impacting the effective growth rate and subsequently altering the doubling time estimate obtained by the Rule of 70. This can result in wider discrepancies between the Rule of 70’s estimate and the actual doubling time.
Conclusion
In conclusion, the Rule of 70 is a powerful yet simple tool that provides a quick and reasonably accurate estimate of the time required to double a quantity at a constant growth rate. Whether it is used to calculate the time it takes for an investment to double due to compound interest, or to estimate the doubling time of a country’s population, it provides a useful ballpark estimate.
However, it is important to remember that the Rule of 70 is only an estimate; it suffers from several limitations which may reduce its accuracy. Hence, it should always be used cautiously and verified with actual data, particularly when making important decisions.
More Resources
Create a free account to unlock this Template
Access and download collection of free Templates to help power your productivity and performance.
Already have an account? Log in
Supercharge your skills with Premium Templates
Take your learning and productivity to the next level with our Premium Templates.
Upgrading to a paid membership gives you access to our extensive collection of plug-and-play Templates designed to power your performance—as well as CFI's full course catalog and accredited Certification Programs.
Already have a Self-Study or Full-Immersion membership? Log in
Access Exclusive Templates
Gain unlimited access to more than 250 productivity Templates, CFI's full course catalog and accredited Certification Programs, hundreds of resources, expert reviews and support, the chance to work with real-world finance and research tools, and more.
Already have a Full-Immersion membership? Log in